Simple Interest and Compound Interest Math Problems for Students

Understanding the concepts of simple interest and compound interest is crucial for students who want to navigate the world of finance and make informed decisions about investments, loans, and savings. Whether you are studying math or simply looking to enhance your financial literacy, this article will provide a comprehensive overview of simple interest and compound interest, their calculations, applications, and their differences. By delving into real-world scenarios and offering practical tips and tricks, this article aims to equip students with the necessary knowledge and skills to solve interest math problems with ease and confidence.

1. Introduction to Simple Interest and Compound Interest

1.1 What is Interest?

Interest is that sneaky little extra bit of money that banks and lenders charge us when we borrow their cash. It's like a service fee for borrowing money or, if you're lucky, a reward for saving it. Whether we realize it or not, interest is a fundamental concept in finance and can have a big impact on our wallets.

1.2 Types of Interest: Simple and Compound

Now, not all interest is created equal. There are two main types: simple interest and compound interest. Simple interest is, well, simple. It's calculated based on the initial amount you borrowed or deposited, known as the principal, and the interest rate. Compound interest, on the other hand, is a bit more complex. It not only takes into account the principal and interest rate but also factors in the interest that accumulates over time. Think of it as interest on top of interest, like a delicious stack of pancakes.

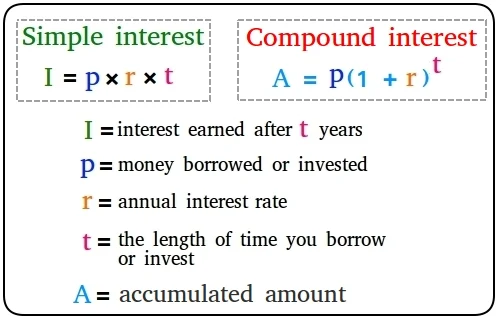
2. Understanding Simple Interest Calculations

2.1 Formula for Simple Interest

To calculate simple interest, you only need three things: the principal, the interest rate, and the time period. The formula is as follows: I = P x R x T, where I is the interest, P is the principal, R is the interest rate, and T is the time period. Easy peasy, right?

2.2 Solving Simple Interest Problems with Given Values

Let's say you borrow $1,000 from your friend at an annual interest rate of 5%. If you only keep the money for 2 years, what will be the interest? Plug the values into the formula: I = 1000 x 0.05 x 2 = $100. See? Piece of cake (or should I say, slice of interest).

2.3 Calculating Time, Principal, or Interest Rate in Simple Interest Problems

Sometimes you're given the interest and other values but need to find the missing piece of the puzzle. Don't worry, it's not a game of hide and seek. With a little rearranging of the formula, you can easily solve for time, principal, or interest rate. Just remember, solving for interest rate can be a bit trickier, so keep an eye out.

3. Exploring Compound Interest Calculations

3.1 Formula for Compound Interest

Now let's dive into the world of compound interest. The formula may look a bit scarier, but don't fret. It's not as complicated as it seems. The formula for compound interest is: A = P(1 + r/n)^(nt), where A is the total amount, P is the principal, r is the interest rate, n is the number of times interest is compounded per year, and t is the number of years.

3.2 Understanding Compounding Periods

When it comes to compound interest, the compounding periods matter. They determine how often interest is calculated and added to the principal. Common compounding periods include annually, semi-annually, quarterly, and monthly. The more frequently interest compounds, the more your money grows. It's like having a mini celebration of interest more often.

3.3 Calculating Compound Interest using Different Compounding Periods

Let's say you deposit $1,000 in a savings account with an annual interest rate of 5% and the bank compounds interest quarterly. After 2 years, how much will you have? Plug the values into the formula, and voila! A = 1000(1 + 0.05/4)^(4*2) = $1,102.52. Compound interest magic at work!

4. Comparing Simple Interest and Compound Interest

4.1 Differences between Simple and Compound Interest

So, what sets simple interest and compound interest apart? Simple interest is linear and only based on the principal and interest rate, while compound interest is exponential and takes into account how interest builds over time. In other words, compound interest can be a real money-multiplying machine.

4.2 Impact of Time and Frequency of Compounding on Interest Earned

Time is money, and when it comes to interest, it's especially true. The longer you leave your money to grow, the more you'll earn in compound interest. Additionally, the frequency of compounding matters. The more often interest compounds, the more it adds up over time. It's like building a snowball that keeps getting bigger as it rolls downhill.

4.3 Evaluating Which Type of Interest is More Beneficial

Now, the million-dollar question (literally): which type of interest is better? Well, it depends on your specific situation and goals. Simple interest is straightforward and may be ideal for short-term loans or simple savings accounts. However, if you're looking for long-term growth and snowballing interest, compound interest is the way to go. It's like having a money-making machine that keeps churning out more and more moolah.

So, whether you love the simplicity of simple interest or the compounding magic of compound interest, understanding these concepts will give you a head start in the world of finance. Just remember to read the fine print, calculate wisely, and watch your money grow. Happy interest-ing!

5. Real-World Applications of Simple and Compound Interest

5.1 Applying Interest Calculations in Banking and Finance

Banking and finance industries heavily rely on interest calculations. Understanding simple and compound interest helps individuals make informed decisions about their savings and loans. For example, banks use compound interest to calculate the interest earned on savings accounts and the interest charged on loans. By knowing how interest is calculated, individuals can compare different banking products and choose the best options for their financial goals.

5.2 Interest in Investments and Savings

Investors and those looking to grow their wealth can benefit from understanding interest calculations. Compound interest plays a crucial role in investments, as it allows for exponential growth over time. By investing their money wisely, individuals can earn returns on both the principal amount and the accumulated interest. This can turn a modest investment into a substantial sum over the long term.

5.3 Understanding the Cost of Borrowing with Interest

Interest calculations are essential for anyone considering borrowing money, whether it's for a mortgage, student loan, or credit card debt. The interest charged on loans increases the overall amount that needs to be repaid. By understanding how interest accumulates over time, individuals can make informed decisions about borrowing and manage their debt effectively.

6. Tips and Tricks for Solving Interest Math Problems

6.1 Identifying Key Information in Interest Problems

When faced with interest math problems, it's crucial to identify the key information provided. Pay attention to the principal amount, interest rate, and time period involved. These details will help you determine which formulas or equations to apply to solve the problem accurately.

6.2 Using Formulas and Equations to Solve Interest Problems

Interest problems often involve using specific formulas and equations. For simple interest, the formula is I = P * r * t, where I represents the interest, P is the principal amount, r is the interest rate, and t is the time period. Compound interest involves more complex formulas, such as A = P * (1 + r/n)^(n*t), where A is the total amount, n is the number of compounding periods, and the other variables have the same meanings as in simple interest. Familiarize yourself with these formulas to solve interest problems efficiently.

6.3 Applying Logical Reasoning to Complex Interest Scenarios

In some cases, interest problems may involve complex scenarios that require logical reasoning. Consider factors such as compounding periods, reinvestment of interest, or changes in interest rates over time. By using logical thinking and considering the context of the problem, you can arrive at the correct solution.

7. Common Mistakes to Avoid in Interest Calculations

7.1 Misinterpreting Given Values in Interest Problems

One common mistake in interest calculations is misinterpreting the given values. Ensure that you correctly identify the principal amount, interest rate, and time period provided in the problem. Pay attention to the units used, such as years or months, and convert them if necessary to ensure accurate calculations.

7.2 Failing to Convert Units or Adjust Compounding Periods

Failing to convert units or adjust compounding periods can lead to incorrect calculations. If the interest rate is given annually but the time period is provided in months, you must convert one of them to match the other. Similarly, when dealing with compounding interest, make sure the frequency of compounding aligns with the given time period.

7.3 Overlooking the Effects of Inflation or Currency Fluctuations

In some cases, interest calculations may overlook the impact of inflation or currency fluctuations. If the interest rate is given in nominal terms, it may not account for the depreciation of money over time. Be mindful of inflation rates and currency fluctuations to make accurate assessments of the true value of interest earned or paid.

8. Practice Exercises to Master Simple and Compound Interest

8.1 Solving Interest Math Problems

To master simple and compound interest, practice solving various interest math problems. Start with basic problems involving simple interest and gradually progress to more complex scenarios with compound interest. By practicing regularly, you'll become comfortable with the formulas, equations, and logical reasoning required to solve interest-related math problems effectively.In conclusion, mastering the concepts of simple interest and compound interest opens up a world of financial possibilities. By understanding how interest is calculated, students can make informed decisions about borrowing, investing, and saving money. Moreover, being aware of the differences between simple and compound interest allows individuals to evaluate which option is more advantageous in various scenarios. Armed with the knowledge gained from this article, students can confidently tackle interest math problems and apply their understanding to real-life situations, setting themselves up for financial success in the future.

Frequently Asked Questions

1. What is the difference between simple interest and compound interest?

Simple interest is calculated based solely on the initial principal amount, while compound interest takes into account both the principal and any accumulated interest. Compound interest can result in higher returns or costs depending on the compounding frequency.

2. How do I calculate simple interest?

Simple interest can be calculated using the formula: Interest = Principal x Rate x Time. Simply multiply the principal amount by the interest rate and the time period (in years) to determine the interest earned or paid.

3. What are the practical applications of simple and compound interest?

Simple and compound interest calculations are widely used in various financial contexts. They are applicable in banking for calculating loan repayments, determining interest earned on savings accounts, and assessing the cost of borrowing. In investments, compound interest helps determine the growth of savings and the potential returns on investments.

4. Are there any shortcuts or tips for solving interest math problems?

Absolutely! Throughout the article, we provide tips and tricks to simplify interest calculations. These include identifying key information in problems, using formulas and equations effectively, and applying logical reasoning to complex scenarios. By practicing these strategies, you can enhance your problem-solving skills and tackle interest math problems with confidence.